Anyway, the basic idea is like this. If you have the real line, or a plane, or 3-dimensional space, or as many dimensions as you want, can you measure every subset of it? I'm just going to talk about the real line (all of the real numbers). If you have an interval, we usually talk about the length of the interval as its measure. But not all subsets are intervals. For instance, the rational numbers are a subset of the real numbers, but they don't have a "length." Is there something like length, but more general, that we can use to measure all subsets?
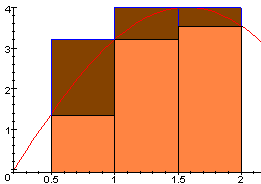 Remember Riemann integration, where you find the area under a curve by approximating with boxes? One way to do that is to measure the boxes that go outside of the curve (the brown ones) and the ones that go inside (the orange ones), then take the limit as you make the boxes narrower, and then see if the two limits are the same, in which case, that limit is the area under the curve. (Intuitively, you can see that if you make the boxes "infinitely narrow," the inside and outside boxes would be the same under a smooth curve like this one. That's what it means to take the limit.)
Remember Riemann integration, where you find the area under a curve by approximating with boxes? One way to do that is to measure the boxes that go outside of the curve (the brown ones) and the ones that go inside (the orange ones), then take the limit as you make the boxes narrower, and then see if the two limits are the same, in which case, that limit is the area under the curve. (Intuitively, you can see that if you make the boxes "infinitely narrow," the inside and outside boxes would be the same under a smooth curve like this one. That's what it means to take the limit.)There is a similar definition of measure, called Jordan Measure. Unfortunately, it doesn't exist for quite a lot of subsets of the real numbers (just like not every function is integrable).
What we really want is a happy kind of measure that satisfies at least the following intuitively obvious conditions:
1. The measure of an interval is the same as its length.
2. If you have two (or more) sets, and they are disjoint (don't overlap), then the measure of their union (both together) should be the sum of their individual measures. (In other words, if you cut something up into pieces, the sum of the sizes (measures) of the pieces should be the same as the size of the original.)
3. If you have two sets, A and B, and A is a subset of B, then the measure of A should be less than or equal to the measure of B. (In other words, if A fits inside of B, then A shouldn't be "larger" under this measure.)
4. It is "translation invariant" - moving a set around (like by adding something to every number in it) doesn't change its measure.
5. The measure of the empty set is 0.
6. Measures are never negative.
What we're studying now in analysis is called Lebesque Measure. Actually, what we have is Lebesque Outer Measure, which is the Lebesque equivalent of the outer box method (the brown boxes above). Here is the difference between Jordan measure and Lebesque measure. In both of them, you are looking at intervals (the 1-dimensional equivalent of boxes; of course when you do this in more dimensions you use boxes or rectangular solids, etc.). In Jordan outer measure, the intervals can't overlap, and they have to be finite in number. In Lebesque outer measure, the intervals CAN overlap, and they can be countably infinite (you can have one for each natural number, going up to infinity). In both cases, you then take the infimum (which is basically the lower limit) of the sum of the lengths of the intervals, for all such sets of intervals.
There is no Lebesque inner measure. Lebesque outer measure exists for every subset of the reals and it has a lot of the nice qualities we want, but it doesn't have criterion 2 (called "additivity") above for all sets. So what they did was, they said, hey, if a set is additive with every other set, then it's "Lebesque measurable." Otherwise, we don't care about it. (Ideally you'd have an even better measure that works perfectly for all sets, but such a thing either doesn't exist or hasn't been figured out yet, as best I'm aware.)
Basically, every kind of set you'd easily think of is Lebesque measurable. Certainly all of the intervals, all singletons, plus sets like the rational numbers are measurable.
Right now, what I'm struggling with is that we have approximately three kadrillion theorems about Lebesque outer measure and about Lebesque measurability, and I'm having a really hard time keeping them all straight, even though I've written out each one with proof and even though I've (several times) made lists of all of them. The idea that I might have to be able to reproduce any or all of these proofs on an exam is terrifying but possibly true. So...that's my own little personal addendum to this otherwise no doubt extremely boring post about math.





















1 comment:
This was brillaint and well-written and really edifying. I'm sure that's surprising, but it's true.
Post a Comment